Research
/Security News
Critical Vulnerability in NestJS Devtools: Localhost RCE via Sandbox Escape
A flawed sandbox in @nestjs/devtools-integration lets attackers run code on your machine via CSRF, leading to full Remote Code Execution (RCE).
Register 3-D point clouds using rotation, translation, and scale transformations.
def Superpose3D(X, # <-- Nx3 array of coords for the "frozen" point cloud
x, # <-- Nx3 array of coords for the "mobile" point cloud
# ---- optional arguments: ----
w = None, # optional weights for the calculation of RMSD
allow_rescale=False, # attempt to rescale mobile point cloud?
report_quaternion=False) # report rotation angle and axis?
Superpose3D() takes two ordered lists (or numpy arrays) of xyz coordinates (of the same length, N) representing points in a point cloud (X and x). Treating them as rigid objects, "Superpose3D()" attempts to superimpose them using rotations, translations, and (optionally) scale transformations in order to minimize the root-mean-squared-distance (RMSD) between corresponding points from either point cloud, where RMSD is defined as:
RMSD = sqrt( (Σ_n w[n] * Σ_i |X[n][i] - (Σ_j c*R[i][j]*x[n][j] + T[i])|^2) / (Σ_n w[n]) )
If w=None, equal weights are used. In that case:
RMSD = sqrt( (Σ_n Σ_i |X[n][i] - (Σ_j c*R[i][j]*x[n][j] + T[i])|^2) / N )
...where:
R = a rotation matrix (a 3x3 numpy array representing the rotation. |R|=1)
T = a translation vector (a 1-D numpy array containing x,y,z displacements)
c = a scalar (a number, 1 by default)
This function returns a 4-tuple containing the optimal values of:
(RMSD, R, T, c)
Note: This function does not attempt to determine which pairs of points from either cloud correspond. Instead, it infers them from the order of the arrays. (It assumes that the i'th point from X corresponds to the i'th point from x.)
If the rotation angle and axis are needed, then set the report_quaternion argument to True. In that case, the function will return this 4-tuple instead:
(RMSD, q, T, c)
...where q is the quaternion corresponding to rotation R, from which the rotation angle and rotation axis can be easily determined.
This function implements a more general variant of the method from this paper: R. Diamond, (1988) "A Note on the Rotational Superposition Problem", Acta Cryst. A44, pp. 211-216.
This version has been augmented slightly to support scale transformations. (I.E. multiplication by scalars. This can be useful for the registration of two different annotated volumetric 3-D images of the same object taken at different magnifications.)
Note that if you enable scale transformations (i.e. if allow_rescale=True), you should be wary if the function returns a negative c value. Negative c values correspond to inversions (reflections). For this reason, if you are using this function to compare the conformations of molecules, you should probably set allow_rescale=False. This will prevent matching a molecule with its stereoisomer.
Note: A C++ version of this repository is available at https://github.com/jewettaij/superpose3d_cpp
FAQs
Diamond's 1988 rotational superposition algorithm (+scale tranforms)
We found that superpose3d demonstrated a healthy version release cadence and project activity because the last version was released less than a year ago. It has 1 open source maintainer collaborating on the project.
Did you know?

Socket for GitHub automatically highlights issues in each pull request and monitors the health of all your open source dependencies. Discover the contents of your packages and block harmful activity before you install or update your dependencies.

Research
/Security News
A flawed sandbox in @nestjs/devtools-integration lets attackers run code on your machine via CSRF, leading to full Remote Code Execution (RCE).
Product
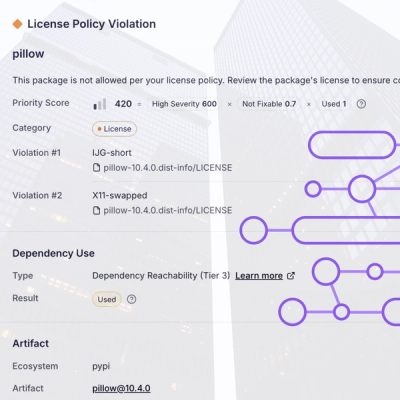
Customize license detection with Socket’s new license overlays: gain control, reduce noise, and handle edge cases with precision.

Product
Socket now supports Rust and Cargo, offering package search for all users and experimental SBOM generation for enterprise projects.