Security News
Opengrep Emerges as Open Source Alternative Amid Semgrep Licensing Controversy
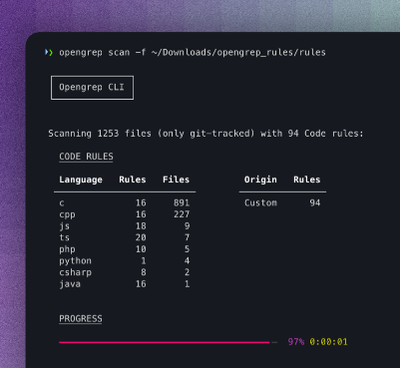
Opengrep forks Semgrep to preserve open source SAST in response to controversial licensing changes.
@id-sdk/geom
Advanced tools
📈 Geometric (planar) math functions
npm install @id-sdk/geom
This library is distributed in ESM format only. It cannot be require()'d from CommonJS.
For more, please read Sindre Sorhus’s FAQ.
import * as geom from '@id-sdk/geom';
import { geomEdgeEqual } from '@id-sdk/geom';
This project is just getting started! 🌱
We're not able to support external contributors at this time, but check back in a bit when things have matured.
# geomEdgeEqual(a: Vec2, b: Vec2): boolean <>
Test whether two given coordinates describe the same edge. Returns true if equal, false if unequal.
geomEdgeEqual([1, 2], [1, 2]); // returns true
geomEdgeEqual([1, 2], [2, 1]); // returns true
# geomRotatePoints(points: Vec2[], angle: number, around: Vec2): Vec2[] <>
Rotate all points counterclockwise around a pivot point by given angle (in radians), without modifying the input points array. Returns an array containing the rotated points.
const points = [[1, 0], [1, 1]];
const around = [0, 0];
geomRotatePoints(points, Math.Pi, around); // returns [[-1, 0], [-1, -1]]
# geomLineIntersection(a: Vec2[], b: Vec2[]): Vec2 | null <>
Return the intersection point of 2 line segments. From https://github.com/pgkelley4/line-segments-intersect This uses the vector cross product approach described here: http://stackoverflow.com/a/565282/786339
// b0
// |
// a0 ---*--- a1
// |
// b1
//
const a = [[0, 0], [10, 0]];
const b = [[5, 5], [5, -5]];
geomLineIntersection(a, b); // returns [5, 0]
# geomPathIntersections(path1: Vec2[], path2: Vec2[]): Vec2[] <>
Return all intersection points of 2 paths.
// b0
// | \
// a0 ---*--*--- a1
// | \
// b1 -- b2
//
const a = [[0, 0], [10, 0]];
const b = [[5, 5], [5, -5], [10, -5], [5, 5]];
geomPathIntersections(a, b); // returns [[5, 0], [7.5, 0]]
# geomPathHasIntersections(path1: Vec2[], path2: Vec2[]): boolean <>
Returns true if paths intersect, false if not.
// b0
// | \
// a0 ---*--*--- a1
// | \
// b1 -- b2
//
const a = [[0, 0], [10, 0]];
const b = [[5, 5], [5, -5], [10, -5], [5, 5]];
geomPathHasIntersections(a, b); // returns true
# geomPointInPolygon(point: Vec2, polygon: Vec2[]): boolean <>
Returns true if point is contained in polygon, false if not.
From https://github.com/substack/point-in-polygon .
Ray-casting algorithm based on http://www.ecse.rpi.edu/Homepages/wrf/Research/Short_Notes/pnpoly.html
// p1 --- p2
// | * |
// p0 --- p3
//
const poly = [[0, 0], [0, 1], [1, 1], [1, 0], [0, 0]];
const point = [0.5, 0.5];
geomPointInPolygon(point, poly); // returns true
# geomPolygonContainsPolygon(outer: Vec2[], inner: Vec2[]): boolean <>
Returns true if every point of inner polygon is contained within outer polygon, false if not.
// o1 -------- o2
// | i1 -- i2 |
// | | | |
// | i0 -- i3 |
// o0 -------- o3
//
const outer = [[0, 0], [0, 3], [3, 3], [3, 0], [0, 0]];
const inner = [[1, 1], [1, 2], [2, 2], [2, 1], [1, 1]];
geomPolygonContainsPolygon(outer, inner); // returns true
# geomPolygonIntersectsPolygon(outer: Vec2[], inner: Vec2[], checkSegments?: boolean): boolean <>
Returns true if any part of inner polygon intersects outer polygon, false if not.
Optionally, pass true to checkSegments option to test each segment (stricter but slower).
// i1 -- i2
// o1 -+------+-- o2
// | | | |
// | | | |
// o0 -+------+-- o3
// i0 -- i3
//
const outer = [[0, 0], [0, 3], [3, 3], [3, 0], [0, 0]];
const inner = [[1, -1], [1, 4], [2, 4], [2, -1], [1, -1]];
geomPolygonIntersectsPolygon(outer, inner, false); // returns false (lax test - points only)
geomPolygonIntersectsPolygon(outer, inner, true); // returns true (strict test - points and segments)
# geomGetSmallestSurroundingRectangle(points: Vec2[]): SSR | null <>
Return the Smallest Surrounding Rectangle for a given set of points, or null for a denerate point set.
See:
// +-- p1 ------ p3
// | |
// p0 ------ p2 --+
//
const points = [[0, -1], [5, 1], [10, -1], [15, 1]];
const ssr = geomGetSmallestSurroundingRectangle(points);
// ssr.poly == [[0, -1], [0, 1], [15, 1], [15, -1], [0, -1]]
// ssr.angle == 0
# geomPathLength(path: Vec2[]): number <>
Return the length of the given path.
// p2
// /
// p0 -- p1
//
const path = [[0, 0], [1, 0], [5, 3]];
geomPathLength(path); // returns 6
# geomViewportNudge(point: Vec2, dimensions: Vec2): Vec2 | null <>
If the given point is at the edge of the padded viewport, return a vector that will nudge the viewport in that direction
# Vec2
An array of two numbers.
[number, number]
# SSR
Smallest Surrounding Rectangle.
An Object containing poly and angle properties. Used as the return value for geomGetSmallestSurroundingRectangle().
{ poly: Vec2, angle: number }
FAQs
Geometric (planar) math functions
We found that @id-sdk/geom demonstrated a not healthy version release cadence and project activity because the last version was released a year ago. It has 2 open source maintainers collaborating on the project.
Did you know?

Socket for GitHub automatically highlights issues in each pull request and monitors the health of all your open source dependencies. Discover the contents of your packages and block harmful activity before you install or update your dependencies.

Security News
Opengrep forks Semgrep to preserve open source SAST in response to controversial licensing changes.

Security News
Critics call the Node.js EOL CVE a misuse of the system, sparking debate over CVE standards and the growing noise in vulnerability databases.

Security News
cURL and Go security teams are publicly rejecting CVSS as flawed for assessing vulnerabilities and are calling for more accurate, context-aware approaches.